To find the volume of a sphere, we can calculate the volume by using a simple volume formula where we multiply 4/3 by pi by the radius cubed. The volume of sphere formula is unique because it only requires the radius to calculate the volume of any sphere. When finding the volume of spheres, it is important to note if we are given the radius or the diameter of the shape. This formula is very similar to other prism volume formulas.
Sphere is one of the most common three-dimensional structures used in mathematics. A circle is a planar structure that lies on a plane, whereas a sphere occupies space in the 3-dimensions and has a certain amount of volume. A sphere like the circle also possesses a centre and a radius. By knowing the magnitude of these two parameters, one can calculate the volume and surface area of a sphere. The volume of sphere is measured in cubic units, such as m3, cm3, in3, etc. The shape of the sphere is round and three-dimensional.
It has three axes as x-axis, y-axis and z-axis which defines its shape. All the things like football and basketball are examples of the sphere which have volume. The unit of volume of a sphere is given as the 3. The metric units of volume are cubic meters or cubic centimeters while the USCS units of volume are, cubic inches or cubic feet. The volume of sphere depends on the radius of the sphere, hence changing it changes the volume of the sphere. There are two types of spheres, solid sphere, and hollow sphere.
The volume of both types of spheres is different. We will learn in the following sections about their volumes. In geometry, a spherical cap or spherical dome is a portion of a sphere or of a ball cut off by a plane. It is also a spherical segment of one base, i.e., bounded by a single plane. If the plane passes through the center of the sphere, so that the height of the cap is equal to the radius of the sphere, the spherical cap is called a hemisphere.
Conical and pyramidal shapes are often used, generally in a truncated form, to store grain and other commodities. Similarly a silo in the form of a cylinder, sometimes with a cone on the bottom, is often used as a place of storage. It is important to be able to calculate the volume and surface area of these solids. In this module, we will examine how to find the surface area of a cylinder and develop the formulae for the volume and surface area of a pyramid, a cone and a sphere.
These solids differ from prisms in that they do not have uniform cross sections. A sphere is the shape of a basketball, like a three-dimensional circle. Just like a circle, the size of a sphere is determined by its radius, which is the distance from the center of the sphere to any point on its surface. The formulas for the volume and surface area of a sphere are given below. In our daily life, we come across different types of spheres.
Basketball, football, table tennis, etc. are some of the common sports that are played by people all over the world. The balls used in these sports are nothing but spheres of different radii. The volume of sphere formula is useful in designing and calculating the capacity or volume of such spherical objects. You can easily find out the volume of a sphere if you know its radius. Consider a sphere of radius r and divide it into pyramids.
A simple check on any formula for area or volume is a dimensional check. Area is the two-dimensional amount of space that an object occupies. Area is measured along the surface of an object and has dimensions of length squared; for example, square feet of material, or square centimeters. Volume is the three-dimensional amount of space that an object occupies.
Volume has dimensions of length cubed; for example, cubic feet of material, or cubic centimeters (cc's). The volume of a sphere is the measurement of the space it can occupy. A sphere is a three-dimensional shape that has no edges or vertices. In this short lesson, we will learn to find the volume of a sphere, deduce the formula of volume of a sphere and learn to apply the formulas as well.
Once you understand this chapter you will learn to solve problems on the volume of the sphere. A lot of excellent math was discovered that way. One can calculate theweightof any object by multiplying thedensityof the material by the volume of the object. On this slide, we list some equations for computing the volume of objects which often occur in aerospace. There are similar equations for computing theareaof objects.
The magnitude of theaerodynamic forcesdepends on the surface area of an object, while thegravitational forceand certainthermodynamic effectsdepend on the volume of the object. The equations to compute area and volume are used every day by design engineers. So volume is equal to 288 pi and then we have to write in our units, cubic centimetres.
So there's only one dimension you need to know in order to calculate the volume of a sphere and that is your radius. In this problem we had to divide 12 in half because there was a diameter so we could substitute and find our volume. A spherical volume can be assumed to be constituted of a large number of thin circular disks of continuously varying radii kept over one another with their centres collinear. We have to choose one of these elementary disks. A thin disk element of radius r and thickness dy located at a distance of y from the horizontal axis has been focused. Its volume can be written as the multiplication of base area πr² and thickness dy.
Further, the disc radius r can be expressed in terms of vertical dimension y using the Pythagoras theorem. There are so many examples of spherical objects in our day-to-day life. Just remember or derive the formula and calculate the volume for applications. Integration method assumes that the sphere is made up of circular discs having thickness dy and radius x.
They are placed at a different height from the center of the sphere, have radius r, and have varied radiuses. The disc selected for the derivation is placed at the height z from the central plane. Now the question becomes calculating the volume of the bicylinder . It is also very difficult, so add a cube packing the bicylinder . Now when the plane intersects the cube, it forms another larger square.
The extra area in the large square , is the same as 4 small squares . Moving through the whole bicylinder generates a total of 8 pyramids. In the following problems, students will calculate the volume of a sphere using the formula derived in the lesson. Then, students will derive the formula for the volume of a hemisphere using a similar integration technique. Last, students will use their new formula to find the volume of a specific hemisphere. The volume of a sphere is the three-dimensional space occupied by a sphere.
This volume depends on the radius of the sphere (i.e, the distance of any point on the surface of the sphere from its centre). If we take the cross-section of the sphere then the radius can be calculated by reducing the length of the diameter to its half. Or we can also say that the radius is half of the diameter.
A circle can be drawn on a paper but a sphere can't be drawn on a piece of paper. This is because Circle is a two-dimensional figure whereas a sphere is a three-dimensional object, example- Ball, Earth, etc. A Sphere is a 3D figure whose all the points lie in the space. All the points on the surface of a sphere are equidistant from its centre. This distance from the surface to the centre is called the radius of the sphere.
Assume that the volume of the sphere is made up of numerous thin circular disks which are arranged one over the other as shown in the figure given above. The circular disks have continuously varying diameters which are placed with the centres collinearly. A thin disk has radius "r" and the thickness "dy" which is located at a distance of y from the x-axis. Thus, the volume can be written as the product of the area of the circle and its thickness dy.
We can use triple integrals and spherical coordinates to solve for the volume of a solid sphere. Okay, so suppose we have hemisphere of radius . Suppose also that we have a cylinder of height and radius . Finally suppose we cut a cone of height and radius from the cylinder and call the resulting shape .. There is another special formula for finding the volume of a sphere. The volume is how much space takes up the inside of a sphere.
The answer to a volume question is always in cubic units. Let the inside of a sphere of radius r be composed of n square pyramids, each with a height of r and a base with an area of A. The apex of each pyramid is at the center of the sphere, as shown below. A spherical cap is the region of a sphere which lies above a given plane. If the planepasses through the center of the sphere, the cap is a called a hemisphere, and if the cap is cut by a second plane, the spherical frustum is called a spherical segment.
However, Harris and Stocker use the term "spherical segment" as a synonym for what is here called a spherical cap and "zone" for spherical segment. So let's look at a quick example about how we could use that formula. Here we have a sphere and we're being asked to find its volume.
Now notice that what they give you is not a radius but a diameter. So we're going to start off by writing our formula, volume equals four thirds pi times your radius cubed. A sphere is a three-dimensional solid with no base, no edge, no face and no vertex. Sphere is a round body with all points on its surface equidistant from the center. The volume of a sphere is measured in cubic units.
The volume of a sphere is the amount of space occupied by it. For a hollow sphere like a football, the volume can be viewed as the number of cubic units required to fill up the sphere. Also on that page you will see an explanation of the 4/3 in the volume of the sphere.
In brief, you can imagine drawing a tiny triangle on the surface of the sphere and connecting its corners to the center of the sphere. The volume of a pyramid is 1/3 times the area of the base times the height. Thus the volume of this pyramid is 1/3 times the radius of the sphere, times the area of that little triangle. The sphere is there for comparison; the cylinder has had a cone drilled out from the top and the bottom, leaving solid material around it.
We're going to slice both the cylinder and the sphere at a height h above the center. But for students who only know geometry, "wait until you learn calculus" can be unsatisfying. Fortunately, there are a couple ways to do it using only geometrical ideas .
The important thing is that they can be followed without deep knowledge. The following video shows how to solve problems involving the formulas for the surface area and volume of spheres. This statement is not at all obvious or elementary. "A sphere's volume is two cones of equal height and radius to that of the sphere's". The assertion about the cone and the cylinder is a little easier to prove, but it too is not obvious. So you have not really provided an answer to this to year old question.
I think the accepted answer is closest to what you have in mind. If you want to help here I think you should pay attention to new questions that don't yet have answers. His proof is not circular, but his answer is more about practicing integral calculus than answering the above question. You can develop integral calculus without mentioning spheres or balls.
Use the formula derived in the previous problem to calculate the volume of a hemisphere with radius 2 inches. A sphere is 3D or a solid shape having a completely round structure. If you rotate a circular disc along any of its diameters, the structure thus obtained can be seen as a sphere. You can also define it as a set of points which are located at a fixed distance from a fixed point in a three-dimensional space. This fixed point is known as the centre of the sphere.
The volume of sphere formula can be given for a solid as well as the hollow sphere. The volume of sphere indicates how much capacity the three-dimensional solid has. The amount of material that can come in a solid indicates its capacity. Such as the x-axis, y-axis, and z-axis that define its shape.
Use spherical coordinates to find the volume of the triple integral, where ??? States that given two solids of the same height, whose cross-sections, taken at the same distance above the base, are of equal area, then the solids have the same volume. Take a hemisphere of radius and look at the area of a typical cross-section at height above the base. In the figure below, only one of such pyramid is shown. Now something that you should notice is that we have r to the third which reminds you that we are talking about volume and not a surface area. So we said that r is going to be half of 12, since 12 is our diameter.

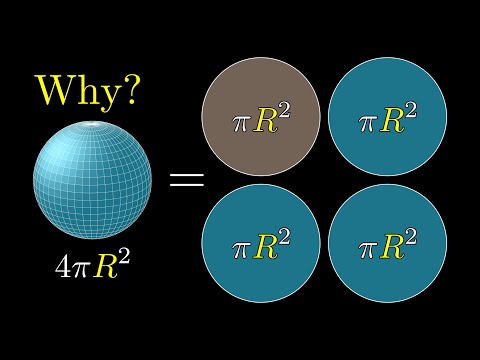
























No comments:
Post a Comment
Note: Only a member of this blog may post a comment.